P.12 Making Calculations from Data

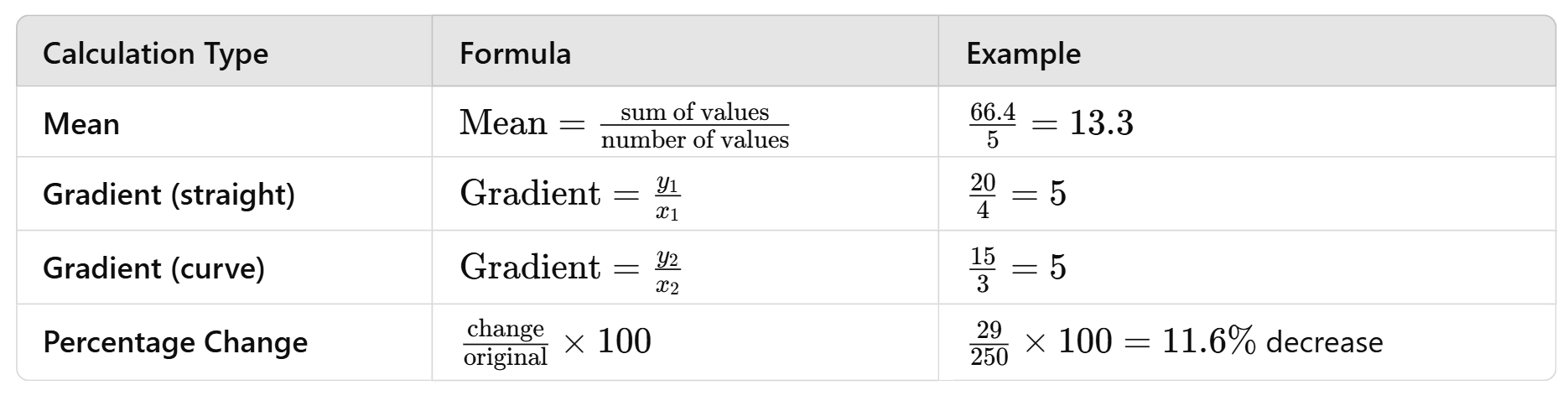
Key Types of Calculations in Experiments

1. Mean Calculation

Purpose: To find the average value from a set of measurements.

Steps:

1. Add all measurements together.
2. Divide by the number of measurements.

Example:

• Given measurements: 12.5 µm, 18.6 µm, 13.2 µm, 10.8 µm, 11.3 µm

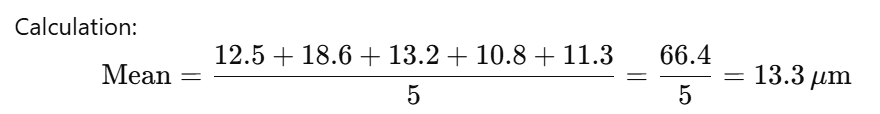
• Decimal Places: Ensure the mean value is presented with the same precision (decimal places) as the original measurements (e.g., 1 decimal place).

1. Gradient Calculation

• Purpose: To determine the rate of change of the dependent variable relative to the independent variable, often representing the rate in scientific data.
• For a Straight Line:
  1. Select two points on the line that are spaced far apart.
  2. Draw a right-angle triangle between the points, marking the changes in x(x₁​) and y(y₁)​.
  3. Use the formula:

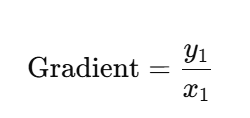
• For a Curved Line:
  1. Draw a tangent to the curve at the point of interest.
  2. Create a right-angle triangle on the tangent line, with marked changes in x(x₂) and y(y₂)​.
  3. Calculate gradient using:

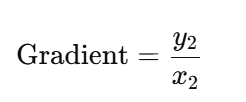
1. Percentage Change

• Purpose: To determine the proportion of change relative to the original value, useful for comparing changes over time or conditions.
• Steps:
  1. Find the difference between the two values (new value – original value).
  2. Divide by the original value.
  3. Multiply by 100 to convert to a percentage.
• Example:
  • Initial mass = 250 g, Final mass = 221 g
  • Change in mass:
    • Change in mass = 250 − 221 = 29g
  • Percentage change:

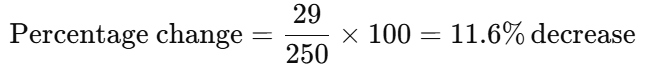
Important Calculation Tips

• Show All Steps: Always display each calculation step for clarity and accuracy.
• Use Appropriate Precision: Match the precision (decimal places) of your calculated results to the original data values.
• Check Units: Ensure that units are consistent throughout calculations and included in the final answer when necessary.

Summary of Key Formulas